Automatic Measurements Of Contact Angle And Curvature In Pore-Space Images

Determining how carbon dioxide (CO2) can be stored securely and safely in underground aquifers, oil and gas flow rate and overall recovery from hydrocarbon reservoirs and shales, the efficiency of gas exchange in leaves in plants and human lungs, the performance of catalysts and fuel cells, how well fabrics resist or soak up water, and the design of water-repellent surfaces, all crucially depend on wettability: how fluid phases interact with solid surfaces within porous structures.

Conventionally, wettability can be inferred through indirect averaged measurements on cm-sized rock samples in the laboratory, such as the Amott and USBM indices, or by studying relative permeability characteristics. At the pore scale, wettability is defined in terms of the contact angle between the fluids residing in the pore space. Moreover, laboratory contact angle measurements are made using smooth mineral surfaces, typically quartz and calcite to represent sandstones and carbonates, respectively.

While wettability has been characterized for external surfaces, we use micron-resolution X-ray three-dimensional imaging to look inside complex porous structures to measure the contact angle and fluid/fluid interface curvature.

In this study, we have developed a novel automated algorithm to measure in situ contact angles and fluid/fluid interface curvature from three-dimensional pore-scale images. The principal idea is to discretize the fluid/fluid and fluid/solid interfaces and define vectors that have a direction perpendicular to these surfaces. The contact angle is found from the dot product of vectors describing the fluid/fluid interface and the solid (rock in this study) surface, where they meet at the three-phase contact line.

We also apply the physical insight that — in equilibrium — the fluid/fluid interfaces should have a uniform curvature. These surfaces are smoothed to increase the accuracy and reliability of the measurements: we apply a volume preserving Gaussian smoothing on all vertices belonging to the generated mesh of the contacted interfaces, which removes the voxelized artifacts from the segmented 3-D image; then, a local uniform curvature smoothing is applied only on the fluid/fluid (oil/brine in our study) interface.

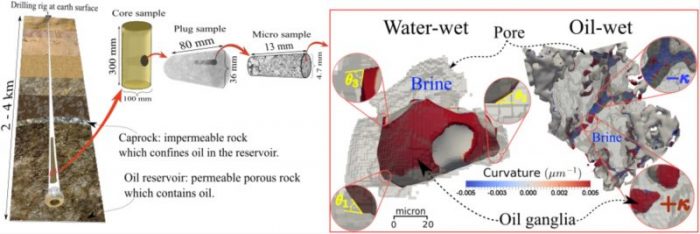
Figure 1. A schematic of wettability and length scales in porous media flows. Right shows images of
oil retained within the pore space of water-wet and oil-wet (or water-repellent) samples. The curvature of the
oil/brine interface is indicated by the colours, while the solid surface in contact with brine is shown in grey. In
a water-wet rock, the oil is trapped in quasi-spherical ganglia with contact angles less than 90◦. For an oil-wet
rock, oil is retained in layers that follow the surface roughness with both positive and negative values of the
curvature κ. This allows the oil to flow to low saturation, facilitating recovery at the km scale (Left). Center
shows how the samples are selected from centimeter-scale cores extracted from a reservoir. Figure republished from PNAS Open Access article: https://www.pnas.org/content/115/36/8901

This novel method has been successfully tested on benchmark synthetic images for which the contact angle and curvature are known analytically and has been applied to a real rock data set. Both a water-wet and a mixed-wet rock have been analyzed. Further, the method has been utilized to study the spatial correlation of the in situ measured distributions of contact angles and oil/brine interface curvatures within reservoir rock samples at subsurface conditions, which originally acquired from a giant oil-producing reservoir in the Middle East.

Measuring in situ contact angles in different types of core samples would allow researchers to define more realistic contact angle distributions, which include natural variability and uncertainty, for reservoir characterization. These distributions could then be used to more accurately predict multiphase fluid flow in pore-scale models. Altered wettability systems that have undergone fluid displacement cycles are likely to have a wide range of contact angles and should not be considered to be uniformly wetting or non-wetting. Furthermore, the curvatures estimated from this algorithm could be used to estimate local capillary pressures.

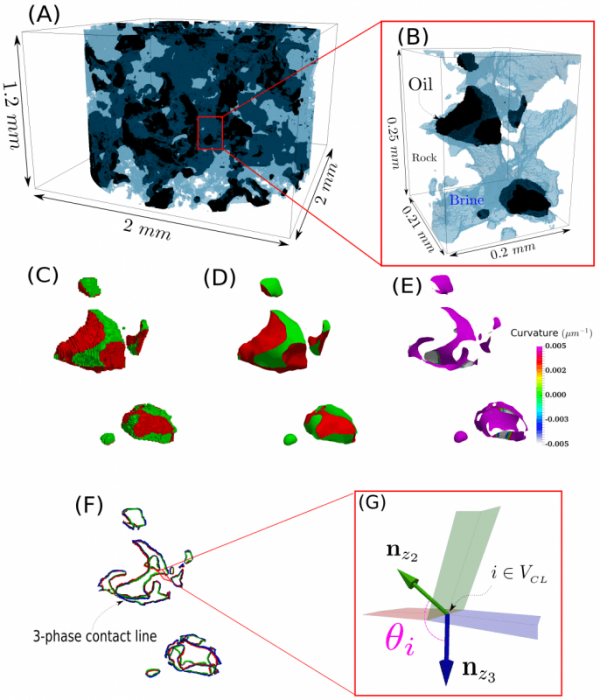
Figure 2. Contact angle and oil/brine interface curvature measurements. (A) Three-dimensional view
of the raw segmented dataset of sample-1 with a voxel size of 2 × 2 × 2 microns. We cropped out a sub-volume from sample-1 in (B) to illustrate the following automatic contact angle measurement workflow. (C) The oil/brine (green) and oil/solid (red) interfaces are identified. (D) The smoothed mesh M that is found after applying both Gaussian and curvature smoothing. (E) The measured oil/brine interface curvature values of all vertices belonging to the oil/brine interface and contact line (i ∈ {VO B , VC L }). (F) The three-phase contact line. (G) Normal vectors are defined on both the oil/brine and brine/rock interfaces for the contact line set, i ∈ VC L. The contact angle is calculated from the dot product of these two normals. Republished from Wiley Open Access article: https://doi.org/10.1029/2017WR022124.

*The codes for measuring contact angle, curvature and roughness are available at: https://github.com/AhmedAlratrout/ContactAngle-Curvature-Roughness

This work was conducted by Ahmed AlRatrout, Ali Q Raeini, Branko Bijeljic, and Martin J Blunt from Imperial College London.

Reference

These findings are described in the articles entitled Automatic measurement of contact angle in pore-space images, recently published in the journal Advances in Water Resources and Spatial Correlation of Contact Angle and Curvature in Pore‐Space Images, recently published in the journal Water Resources Research.

AlRatrout, A., Blunt, M., & Bijeljic, B. (2018b). Wettability in complex porous materials, the mixed-wet state, and its relationship to surface roughness. Proceedings of the National Academy of Sciences, 115 (36), 8901-8906. (USA) doi: 10.1073/pnas.1803734115