Making Sense Of Quantum Mechanics: Geometric Quantum Theory

The theory of quantum mechanics lies at the basis of our modern understanding of how microscopic particles such as electrons and atomic nuclei behave. Despite this fact, controversy on what it actually says about the physical world has accompanied the further development of the theory and its various off-springs up to today.

Perhaps the main question in the debate on the interpretation of quantum mechanics has been determining the physical meaning of the “wave function,” the central mathematical object in the theory. In one of the simplest quantum mechanical models, the 1-particle Schrödinger theory, this wave function is a mathematical function assigning to each point in space and time a (complex) number. If one knows the initial value of the wave function at each point in space, then the wave function at some later time can in principle be determined by solving the Schrödinger equation.

How to use the wave function

In spite of the addressed controversy on its interpretation, quantum mechanics does give a universally-agreed-upon answer to this question: First, the wave function is used to compute the probability to find the particle in a given region of space. Second, the wave function determines the expectation values of so-called observables of the particle – as, for instance, its energy, its velocity and, of course, its position. That is, in order to apply the theory sensibly, one considers a multitude of repetitions of the same experiment.

To be more specific, think of the case of shooting an electron through an experimental apparatus and letting it hit a detector screen, as is done in the famous double-slit-experiment. As explained, one repeats the experiment under the same circumstances until enough data has been gathered. The theory itself is relevant when one wants to know the final statistics (where the electrons tend to hit on the screen) from the initial statistics (where the electrons started out) without having done the experiment.

More precisely, one measures the initial position and velocity of each electron in the ensemble (setting the clock anew each time the experiment starts over) and can determine an initial wave function from that data. Again, solving the Schrödinger equation for this initial wave function and apparatus yields the wave function at any later time. This can then be translated back into the pattern on the screen, which emerges when plotting all of the individual impacts on the screen (see figure). It is important to emphasize, however, that it is impossible to predict, where an individual particle will appear on the screen. Rather, the wave function predicts the final pattern or, better to say, the probability to find a particle in the ensemble within a certain region on the detector.

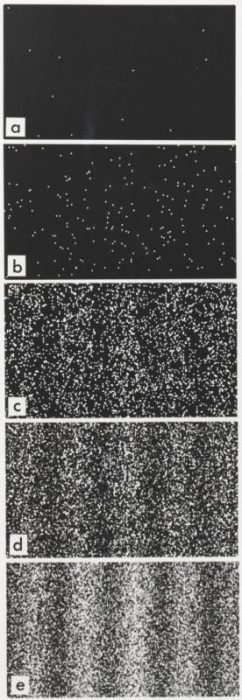
Figure: Results of a double-slit-experiment performed by Dr. Tonomura showing the build-up of an interference pattern of single electrons. Numbers of electrons are 11 (a), 200 (b), 6000 (c), 40000 (d), 140000 (e). This picture was licensed by Belsazar under the GNU Free Documentation License.

The Controversy

The above discussion suggests that quantum mechanics is a purely statistical theory, i.e. one only used to compute the probabilities of the whereabouts of particles and expectation values of the respective observables. Yet there are many physicists, who do not subscribe to this view, also known as the ensemble interpretation. In most interpretations of quantum mechanics, the wave function is given a meaning going beyond its purely statistical interpretation. The book by J. Baggot shall be recommended here for further reading.

For instance, in the Copenhagen interpretation, the wave function describes an actual wave propagating through space and time, which collapses into a pointlike particle once a measurement takes place. This is still the orthodox point of view, shared by the largest portion of physicists in the field, but we are far from reaching any kind of consensus.

The ensemble interpretation is minimalist in the sense that it makes the theory usable without asserting much on “the nature of reality.” Yet, taking it to its logical conclusions, as the author has done in a recent article, is in itself a challenge to the orthodoxy.

The Findings

In 1926, the physicist E. Madelung rewrote the Schrödinger equation in a manner that exposed how the theory is linked to Newtonian (continuum) mechanics, obtaining what is today known as the Madelung equations. In the 1950s David Bohm employed this to develop his own interpretation of quantum mechanics, which is still an active area of research.

If, however, the ensemble interpretation is applied to the Madelung equations, as the author has done in his research, the Madelung equations show that the reason for this unpredictability is the universal presence of a noise. This “Bohm noise” acts on each individual particle in the ensemble and it can be proven that it is only relevant for particles of sufficiently small mass – explaining why the relevant quantum effects are not noticeable on our everyday scales. The physical origin of the Bohm noise is still unknown, one possible explanation is the existence of a gravitational background radiation.

Further, this ansatz questions the established status of the uncertainty principle: When quantum mechanics is taken as a purely statistical theory, the mathematical relation corresponding to the “principle” is one of statistical nature, not one that refers to any individual particle. Therefore, this approach to the Schrödinger theory does not put an upper bound on the possible precision of simultaneous measurement of position and velocity of any individual particle, even though experimental limitations may do this in practice. This, in turn, allows for the physical existence of particle paths – a finding fitting well into the recent discovery of macroscopic quantum analogs.

Finally, it was shown that an ensemble interpretation of the Madelung equations allows for the experimental reconciliation of the Schrödinger theory with the established theory of probability. To be more specific, it has been proven that the fundamental change of the 1-particle Schrödinger theory to accord with the rules of standard probability theory yields the same predictions as standard quantum mechanics in the experimentally relevant cases, but the change also leads to different predictions in experimentally less accessible instances.

Since the Madelung equations are also more suitable to study quantum dynamics with geometric constraints (think e.g. of an electron restricted to move on the surface of a sphere) and this geometric-analytic approach of studying the Schrödinger theory was inspired by the theory of geometric quantization, the author has termed the adapted theory geometric quantum theory.

These findings are described in the article entitled The Madelung Picture as a Foundation of Geometric Quantum Theory, recently published in the journal Foundations of Physics. This work was conducted by Maik Reddiger while study at the Technische Universität Berlin.